
①We can clearly see the dotted points on the graph indicate what the f(x) and x are.į(0)=g(0)≈ 5.2 !we dont need to know the exact value for it, but all we need to know is that f(0)=g(0) would be on the same point. Essentially its just reversed of Sal does in the video. My ways of doing is first find the points we can refer to on the graph, f(x)=?, then slowly figure out the corresponding points of g(x). My method(i feel more comfortable with): Unlike how Sal does in the video, where he figures out x of g(x) right on the get-go. ( at least thats how it looks to me) Which is like putting cart before horses. But in this particular lesson, his method just seems straight out copy from textbook, he knows the answer from the start, and use the answer to explain how you solve it. Not trying to disrespect Sal, he is a amazing teacher. But how do you find a appropriate x at first glance, so that we can use that to refer points on graph? What if the question gives us more complicated function like cube root or x to fifth power instead of 2x? There's no way you can figure them out that fast. So two times x, 2⋅2=4, f(4) which we can see on the graph is equal to 0. Yes, we know the relationship between the two functions is g(x)=f(2 x), which means two times of x would give us the f's point which we can go to the graph to refer it and find the corresponding point on y-axis f(2x). He just put 0 2 -2 (x from g(x) seemingly out of nowhere. Sal uses table to solve it, but the part that confused me is how he picks the x in the table.
#Horizontal compression rational functions free#
Feel free to correct me if I have made any mistakes. So came up the method similar to Sal's but uses different angle to see the problem, and I also made it more understandable for myself. I think I would have gotten more confused if I used his method to do the problems in the exercise. But I still think Sal's way of explanation was a bit rough(especially problem1).
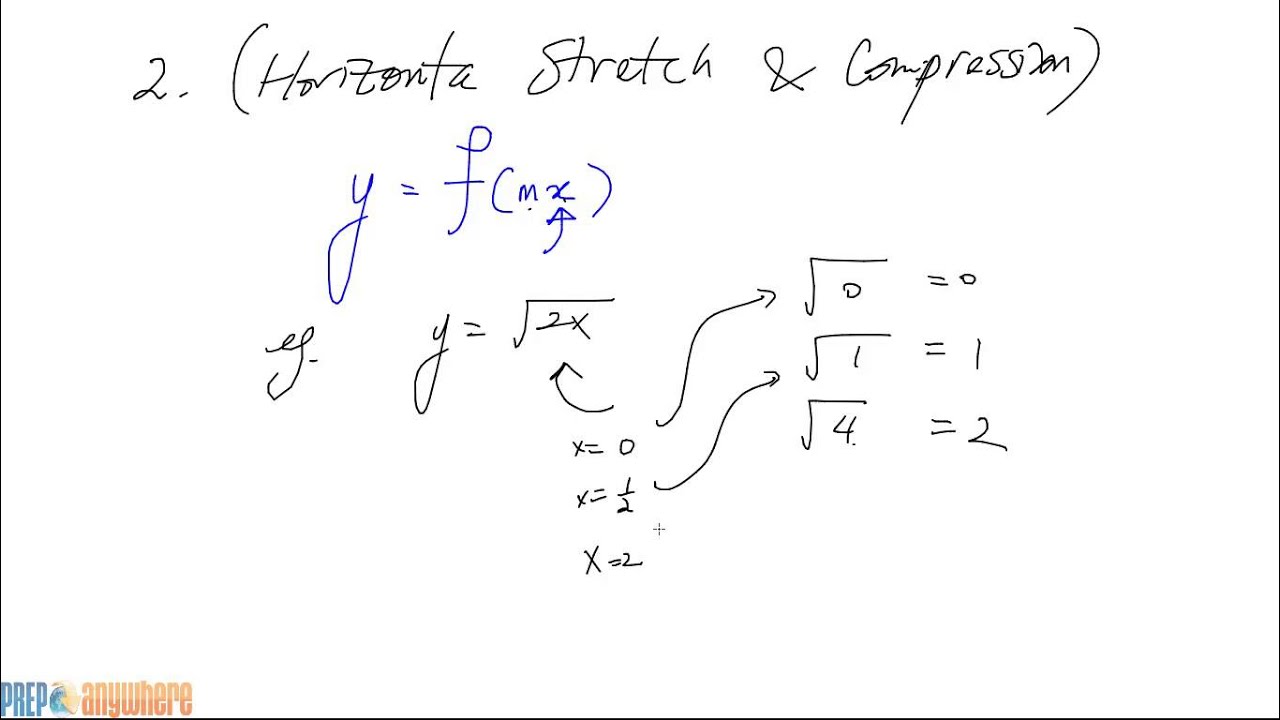
I was so confused when I first watched this video, then today I attempted to understand this video once again. I wanna share this method which I came up after rewatching the video over and over again.(got the idea from Sal's vid)


 0 kommentar(er)
0 kommentar(er)
